correct answer is: a) ₹2000.

Explanation

\large\frac{3}{5} th part of the property value is ₹4200
\therefore 1 th part of the property value is =\left(4200\div\large\frac{3}{5}\right)
\therefore\large\frac{2}{7} th part of the property value is =\left(4200\div\large\frac{3}{5}\times\frac{2}{7}\right)
\rightarrow\left(4200\times\large\frac{5}{3}\times\frac{2}{7}\right)
\rightarrow ₹2000
Ans: The value of \large\frac{2}{7} th part of the property ₹2000.

Another way, Rules of Three Method

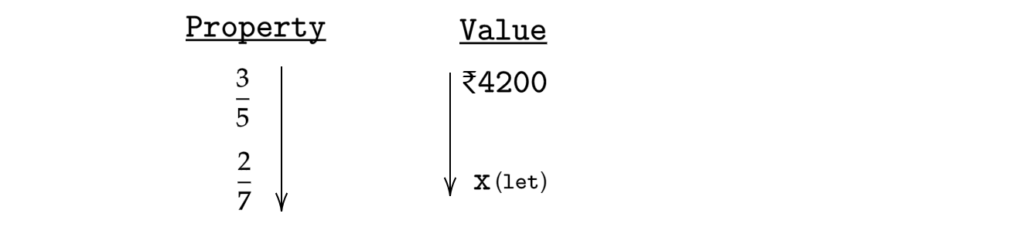
Property and Value are Directly Proportional.
\therefore\large\frac35\;:\normalsize\;4200\;:\;:\;\large\frac27\;:\;x
\rightarrow\large\frac35\;\times\;\frac1{4200}\;=\;\frac27\;\times\;\frac1x
\rightarrow\large\frac15\;\times\;\frac1{1400}\;=\;\frac2{7x}
\rightarrow\large\frac1{7000}\;=\;\frac2{7x}
\rightarrow 7x=7000\times2
\rightarrow x=\large\frac{7000\times2}7
\rightarrow x=2000
Ans: \large\frac{2}{7} th part of the property value is ₹2000.

These particular math questions can also be asked in the following ways:

1. If the value of 3/5th part of a property is ₹4200, what is the value of 2/7th part of the property?
2. How much is the value of 2/7th part of a property if the value of 3/5th part is ₹4200?
3. Given that the value of 3/5th part of a property is ₹4200, what is the value of 2/7th part of the same property?
4. Given that the value of 3/5th part of a property is ₹4200, what is the value of 2/7th part of the same property?